Bathroom Vanity, Small, Modern, 24", Brown Oak, Free Standing
Nameeks DERIN49
Highlights
Overview
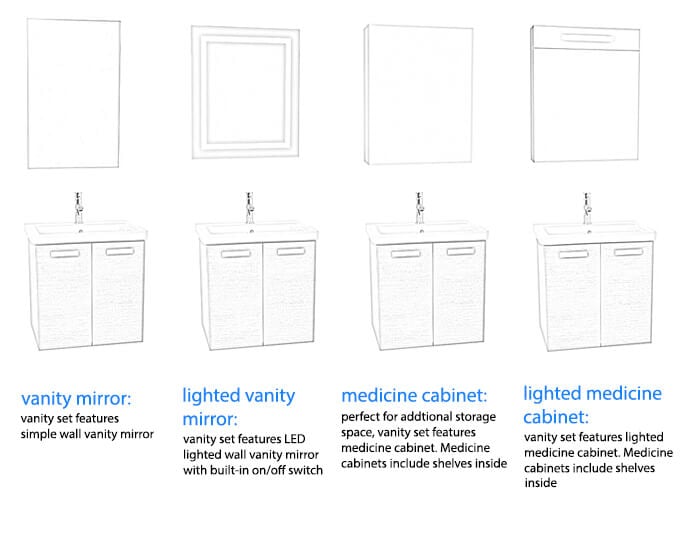
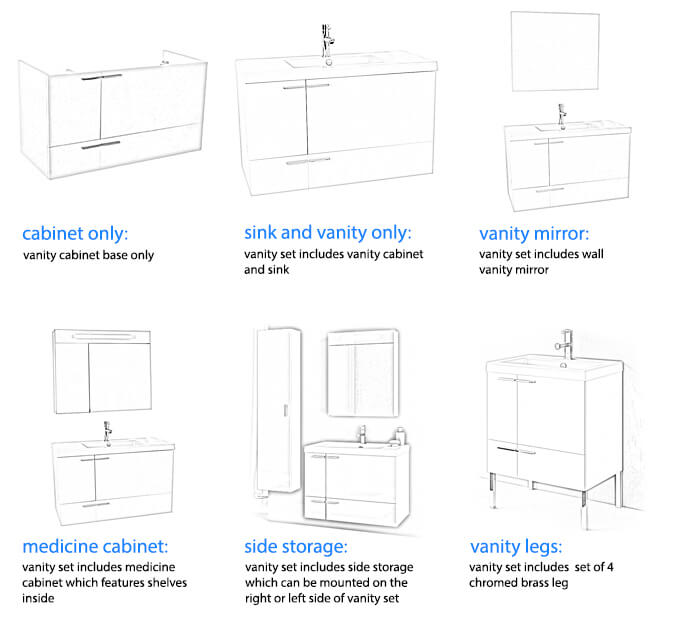
What's Included?
Description
This modern floor standing bathroom vanity set was designed by high-end brand Nameek's. Inspired by modern and contemporary style bathroom design, this bathroom vanity set comes with a beautiful matte black ceramic sink that perfectly fits into a vanity cabinet with two spacious drawers. Ceramic sink features left aligned basin which allows for more counter space on the right side of the sink. Vanity cabinet is made of durable engineered wood and features two soft-closing drawers with sleek black drawer handles. Vanity cabinet attaches on top of a beautiful black base with four vanity feet. This vanity set is part of the Derin collection. Make a bold statement in your bathroom now with this modern bathroom vanity set.
Product Details
- Width: 23.6" Depth: 17.32" Height: 34.65"
- Vanity cabinet made of engineered wood
- Matte black ceramic sink
- Cabinet features water resistant exterior panels
- Vanity cabinet in brown oak finish
- Black vanity cabinet feet
- Black vanity cabinet handles
- Cabinet features 2 soft-closing drawers
- Faucet not included
- Perfect for modern bathrooms
- Ceramic sink features counter space
- Includes manufacturer 1 year warranty
- Wood grain and color variations are to be expected
Technical Specifications
| Download: | |
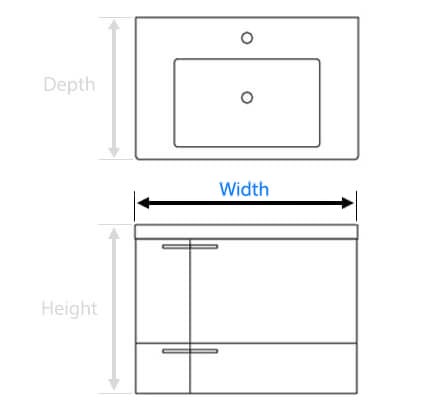
| Overall Width: |
 23.6 Inches
|
| Overall Depth: |
17.32 Inches
|
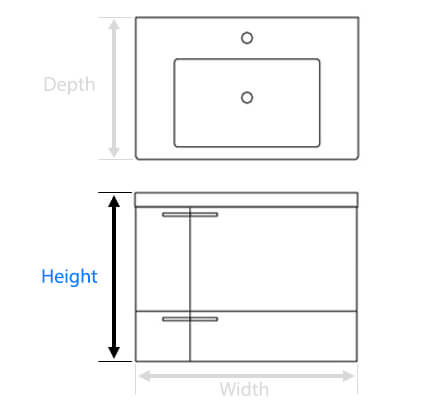
| Overall Height: |
34.65 Inches
|
| Weight: |
140 Lbs
|
| Vanity Cabinet: |
Width: 23.23" Depth: 16.93" Height: 19.69"
|
| Bathroom Sink: |
Basin Depth: 3.54" Basin Width: 12.2" Basin Length: 13.39"
Overall Length: 23.62" Overall Width: 17.32" Overall Height: 5.51" Download Specs |
| Vanity Foot: |
Width: 23.23" Depth: 16.93" Height: 9.45"
|
What's In the Box
3 items
23 Inch Wall Mount Brown Oak Bathroom Vanity Cabinet With Black Handles
Black Ceramic Wall Mounted or Vessel Sink With Counter Space
Scarabeo 5114-49-One Hole
Matte Black Vanity Cabinet Feet
Features
| Sink Finish: |
Matte Black
|
| Top Material: |
Ceramic
|
| Top Finish: |
Matte Black
|
| Color: |
Brown Oak
|
| Style: |
Contemporary, Modern
|
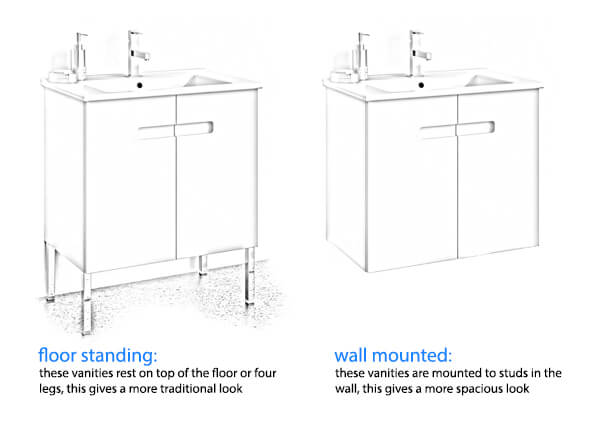
| Installation: |
Floor Standing
|
| Cabinet Material: |
Engineered Wood
|
| Cabinet Finish: |
Brown Oak
|
| Number Of Sinks: |
Single
|
| Pieces Included: |
Sink, Vanity, Sink and Vanity Only, Vanity Cabinet Feet
|
| Handle Finish: |
Matte Black
|
| Application: |
Bathroom
|
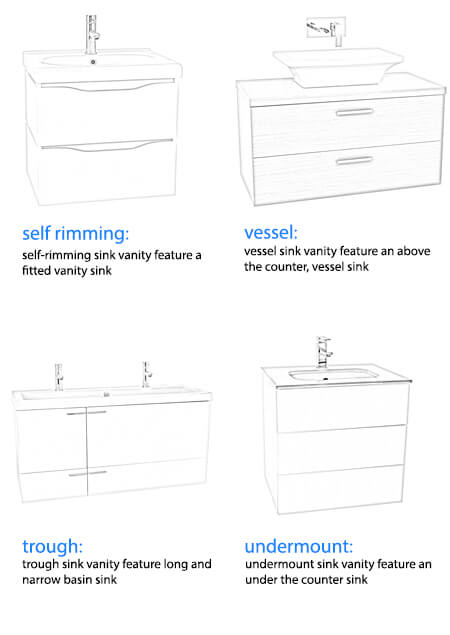
| Sink Type: |
Self Rimming
|
| Mirror Type: |
No Mirror
|
| Features: |
Sink and Vanity Only, Vanity Legs, With Counter Space
|
| Sink Material: |
Ceramic
|
| Type: |
Single
|
| Shape: |
Rectangular
|
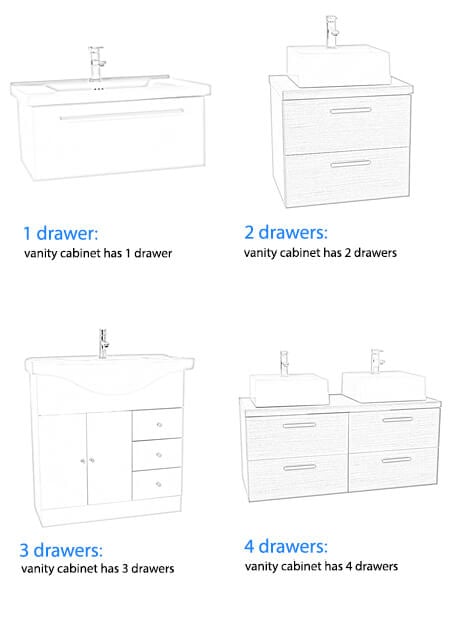
| Drawers: |
2 Drawers
|
| Number Of Pieces: |
3 Piece
|
| Vanity Finish: |
Brown Oak
|
| Vanity Material: |
Engineered Wood
|
| Additional Note: |
Set includes single faucet hole sink. If you would like a no hole or 8 in. widespread configuration, please call in to customize your order.
|
| Soft Close Drawer(s): |
Yes
|
| Cabinet Fully Assembled: |
Yes
|
More Information
| Product Name: | Bathroom Vanity, Small, Modern, 24", Brown Oak, Free Standing |
| SKU: | Nameeks DERIN49 |
| Type: | |
| Sub Type: | |
| Collection Name: | |
| Brand Name: | |
| Country of Origin: | Europe |
| Categories: | |
| California Prop 65: |
Yes
|
Availability & Shipping
| Availability: | In Stock. |
| Shipping Method: | Ships with FedEx. |
Items Included
| Vanity Cabinet: |
23 Inch Wall Mount Brown Oak Bathroom Vanity Cabinet With Black Handles.
Nameeks DER01-01
|
| Bathroom Sink: |
Black Ceramic Wall Mounted or Vessel Sink With Counter Space.
Scarabeo 5114-49-One Hole
|
| Vanity Foot: |
Matte Black Vanity Cabinet Feet.
Nameeks NLG01
|
Product Q/A's
6 questions
?
How do you remove the draws to install the plumbingA
Please see attached video of how to remove the drawers from the vanity.
https://www.youtube.com/watch?v=G2Th2B0Mfq0?
does the sink have a flat bottom?A
The bottom of the sink is slightly sloped to allow the water to drain.?
What is the countertop and sink made ofA
The top is a matte black ceramic sink with countertop space.?
Are the faucet and drain closure included??
Is the back open or does it have a back side?A
The back of the vanity cabinet is open; please see the technical specification page to see the specifications of the cabinet.
Do you have a question about this product?
Nameeks Brand Information
Nameek's is a leading designer, developer, and marketer of luxury home & bath products. In cooperation with top European manufacturers, Nameek's choice of designs has become extremely diversified. Nameek's experience in the plumbing industry spans 30 years and today, Nameek's distributes its products throughout the world.
Customers Also Viewed
Related Searches
